Seeing the Volume of Rectilinear Solids

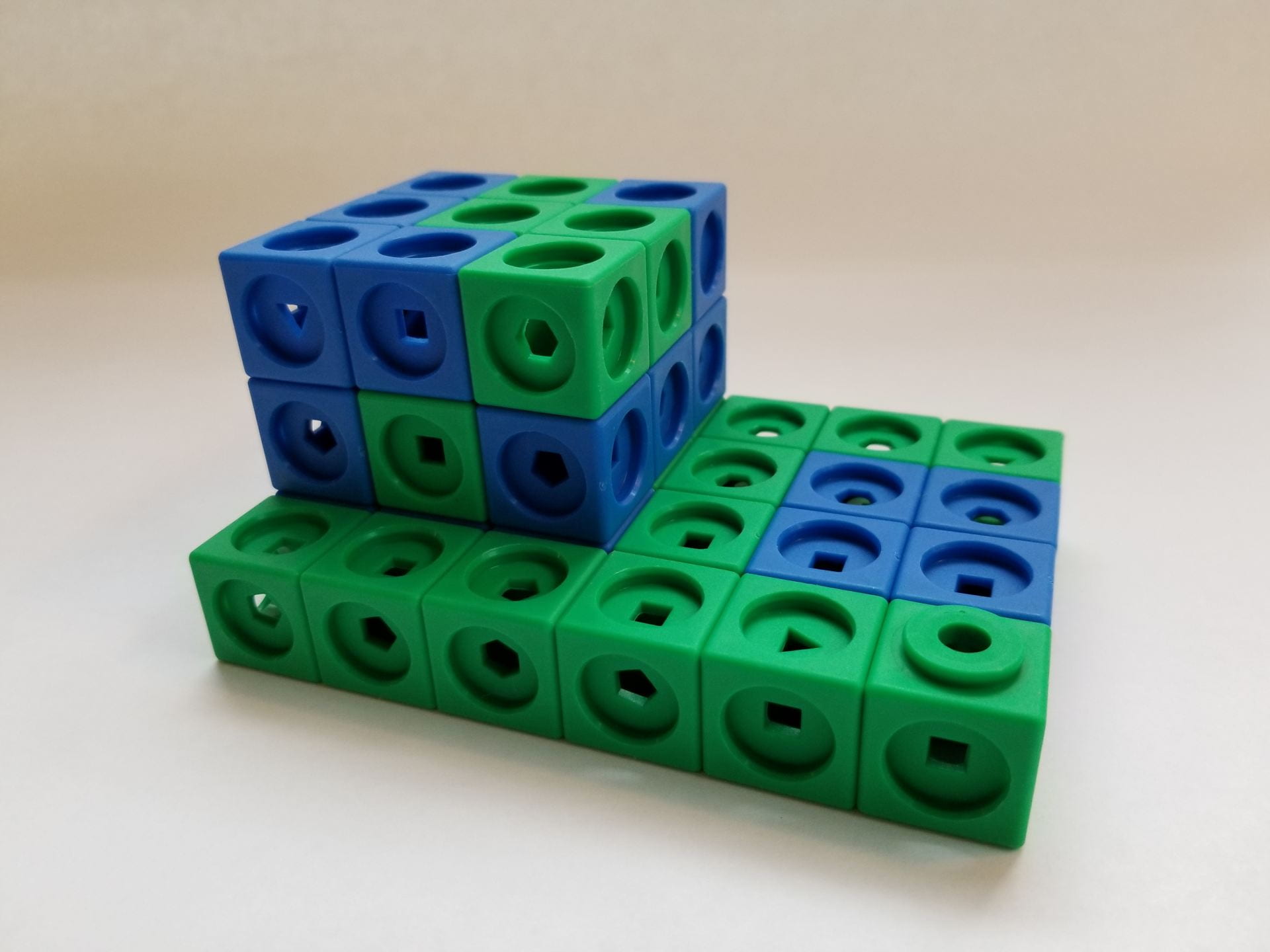
Last fall, we introduced some new images of rectangular solids built with snap cubes where we asked, How many cubes are in this solid? Figuring that out involves counting, spatial reasoning, and multiplicative thinking. One of the biggest mathematical challenges is imagining and counting cubes you cannot see. With this week’s image, we extend that idea to images of what are called rectilinear solids, or three-dimensional figures that are composed of rectangular prisms like the one you see here.

Ask yourself, How many cubes are in this solid? What does you mind do to try to solve this problem? What challenges do you see? How do you deal with them? One key strategy you might have tried is decomposing the figuring into parts you can count separately. What ways could you decompose this solid into parts that are easier to count? Here are just a few ways you and your students might come up with:

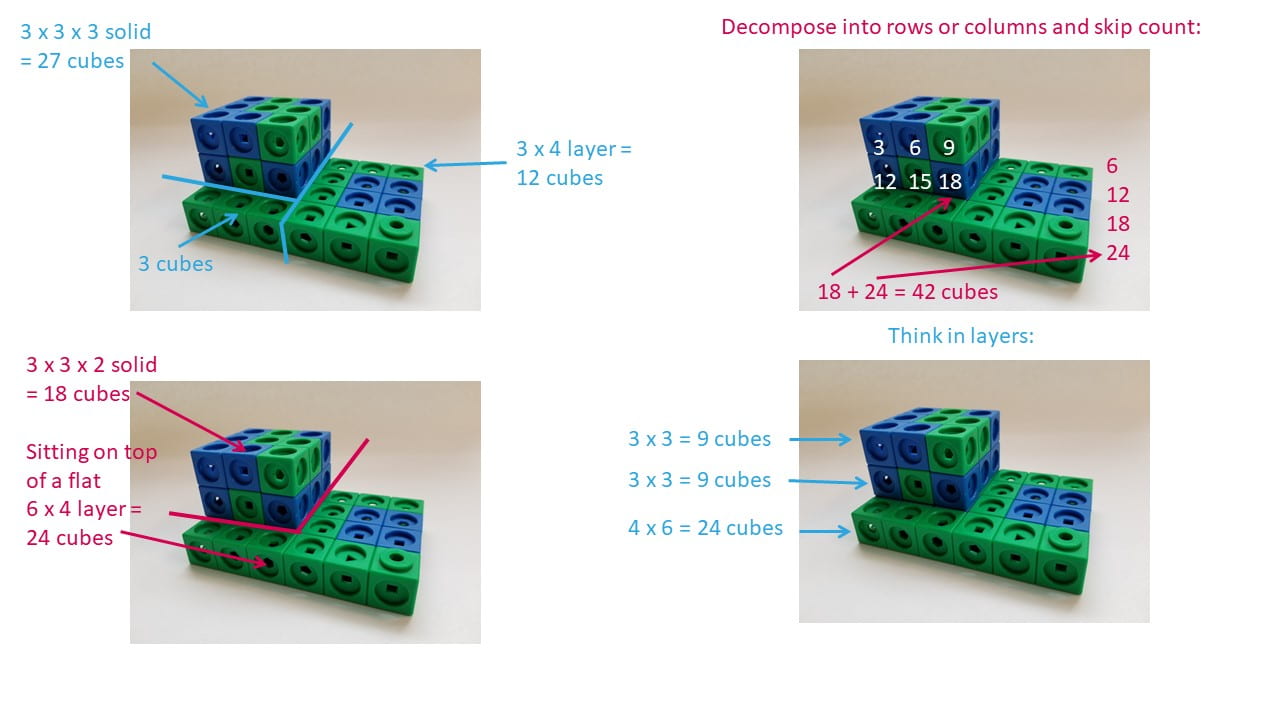
Notice that students might draw on multiplicative thinking in the form of skip counting, or think in layers of arrays, in ways that parallel the way we think about area. Other students might have experience with volume of rectangular solids and think in three dimensions by creating smaller rectangular solids. There are many ways to see how this figure might be composed. Importantly, all of these ways imagine and account for cubes under and behind the cubes you can see. This is challenging mathematical work that integrates big ideas about volume, multiplicative thinking, and composing and decomposing shapes, but even younger students who have not yet been formally exposed to volume can investigate how many cubes are in this figure.

If you have access to cubes, invite students to build the figure to develop or test their thinking. With the solid in hand, they can physically decompose the shape, count the cubes, rotate the figure, and see it from new perspectives. You might also challenge students with our extension task: What other solids could you build with the same number of cubes? All the resulting figures would have the same volume, but some may appear “bigger” than others. Why? This is another avenue to explore measurement, dimensions, and comparison.

And we invite you to follow us on Twitter! Tweet us the fascinating ideas you students have about our activities or how you’re trying these activities in your space. We can’t wait to hear from you!

To multiplicity, cheers!

Jen Munson and the multiplicity lab group