Image of the Week: Exploring Domino Patterns

Exploring Domino Patterns

We love dominoes! There is so much you can do with dominoes around number, pattern, and structure. Try it yourself. Have a look at our Image of the Week: What patterns do you see?

You might have looked across the tops and bottom rows of dots, or seen each domino for its sum, or compared the top and bottom values, or even looked diagonally. Here are just a few of the patterns you might have seen inside this series of dominoes:

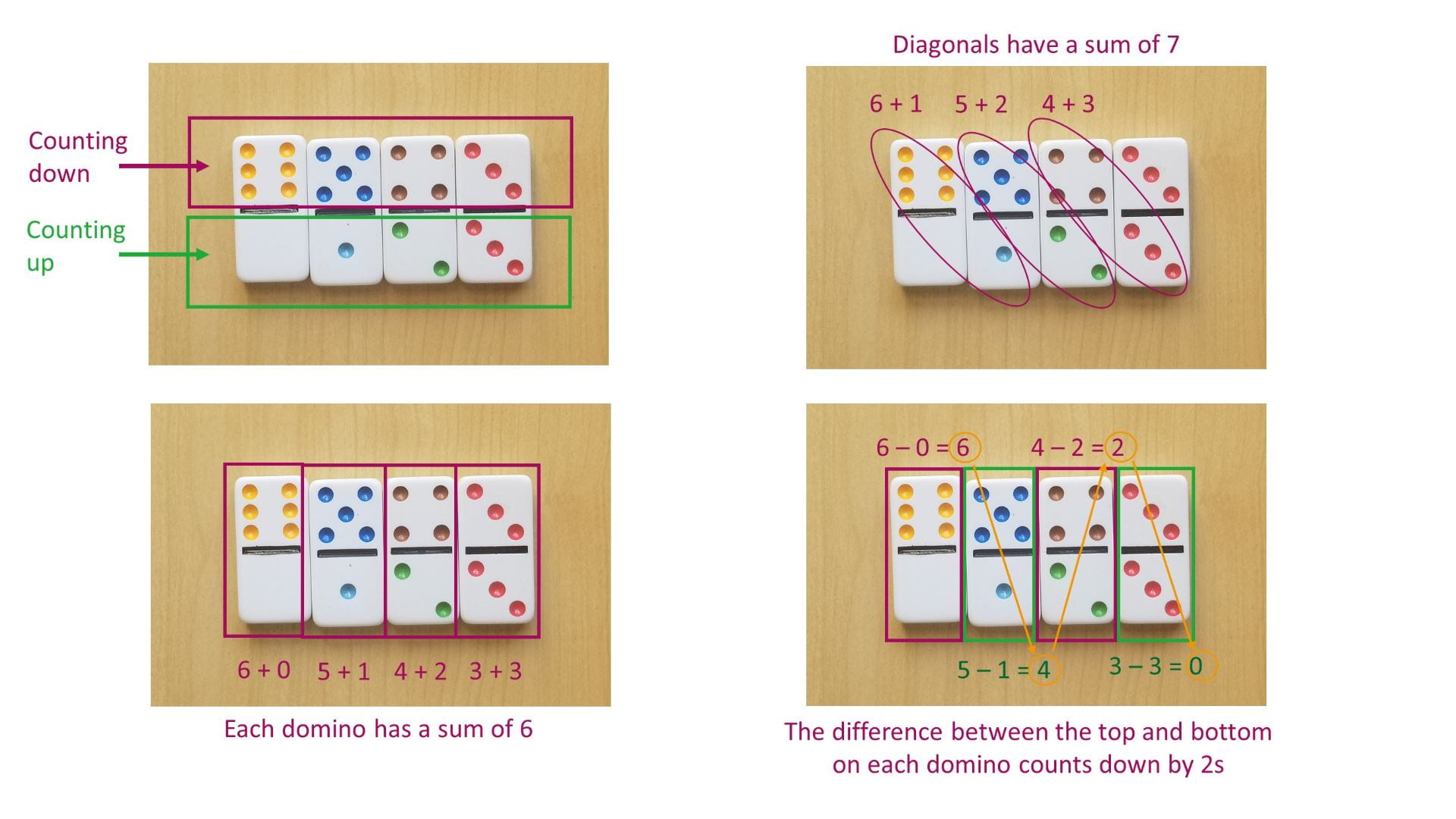
Simply inviting children to look for patterns in this domino image – or one of the other domino pattern images on our website – can open up dozens or diverse observations. Every student can enter a task like this, and the longer you dwell on these patterns, the more students will notice. Why does this matter? Pattern-seeking and looking for structure are at the heart of what it means to do mathematics. Too often when we engage students in thinking about patterns, the patterns are simplistic or we have a specific pattern we want students to notice. Opening up pattern-seeking allows students to see that, in patterned spaces, there are likely to be many layered patterns and many ways to describe those patterns.

These images invite several follow-up questions:

• How could we describe the pattern you noticed?
• How could we represent the patterns you noticed?
• Why does this pattern exist?
• What do you predict will come next in your pattern? Why?
• What domino makes sense to add to the left or right of this pattern? Why?
• Would you pattern continue if we added another domino? How do you know?
• If we extended this pattern to include 10 (or 15 or 50) dominoes, what would you predict would happen to the patterns you noticed? What domino would be the 10^th (or 15^th or 50^th) domino in the sequence?

You can see that these questions take what is a simple series of dominoes and extend the exploration well up into middle school by asking children to predict from and, ultimately, generalize the patterns they see. Thinking about how to extend to the left or right may push the dominoes or students’ patterns beyond the counting numbers into integers. (What would a domino with a 7 on top and -1 on the bottom look like?)

Whether you have very young students, whose observations focus on the counting sequence and sums of the individual dominoes, or much older students ready to tackle describing their patterns as algebraically, exploring domino patterns will give you and your students lots to talk about.

To multiplicity, cheers!

Jen Munson and the multiplicity lab group