Image of the Week: Conservation in Counting Sets
March 20, 2022Conservation in Counting Sets
If you teach young children, then you likely know that counting is complex work. Counting involves coordinating three different concepts:
- Understanding the counting sequence (one, two, three, four,…): There is a fixed counting sequence with no repeating number names.
- One-to-one correspondence: Each object is matched to exactly one number name.
- Cardinality: The last number named when counting a set describes the total number of objects in the set.
If you’ve watched very young children count, you will likely have seen these concepts in development. Building understanding of the counting sequence, one-to-one correspondence for increasingly large sets, and the cardinality principle takes thousands of exposures to counting over many years. This is why we at multiplicity lab offer so many activities specifically devoted to counting ideas through the question, How many do you see? We want to give students opportunities to count lots of different groups, and also, the same group many times. Counting and recounting the same group of objects raises a fourth counting concept that we want to talk about today: conservation.
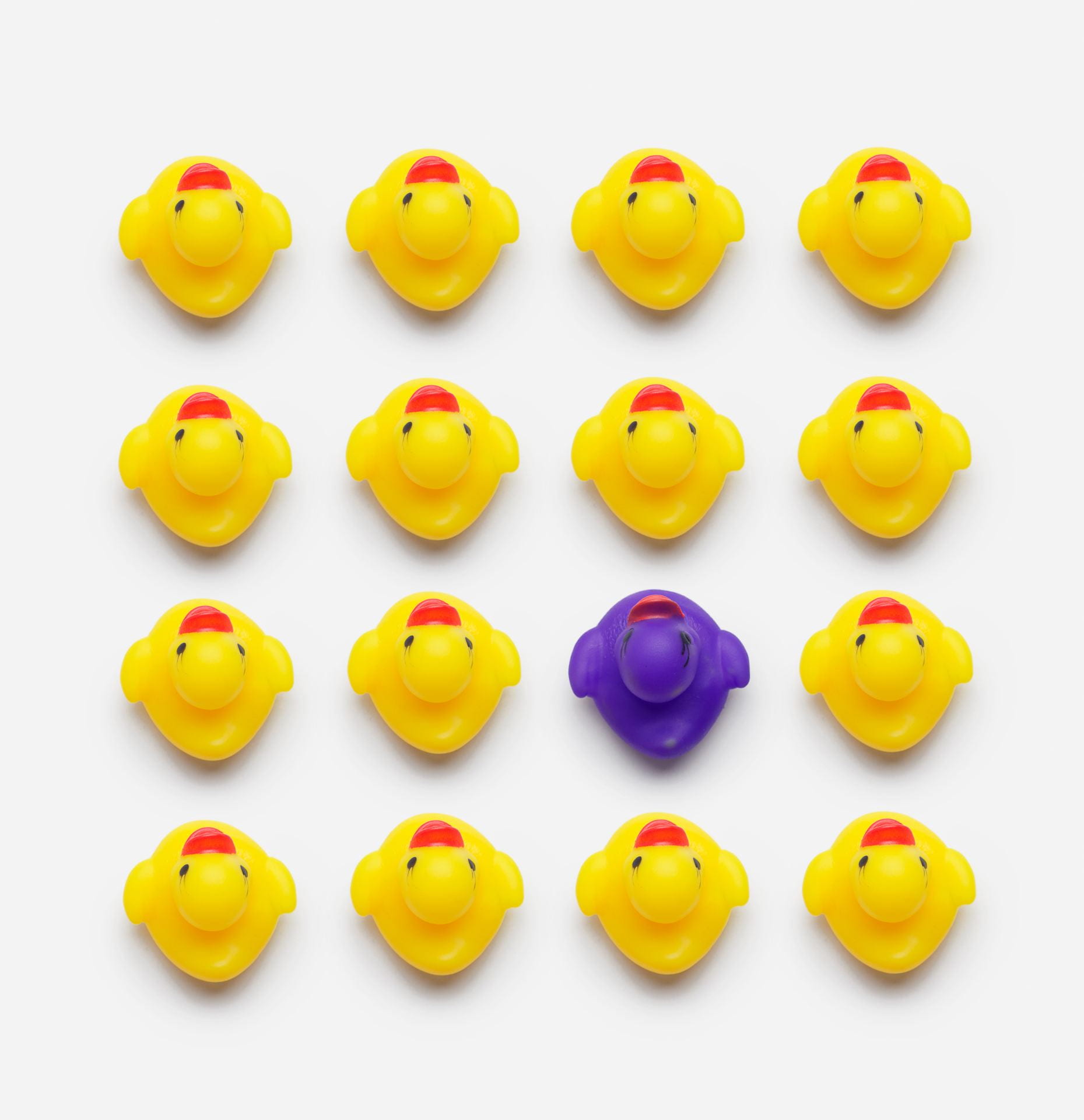
Conservation means that every time you count the same set, no matter how you count them, you get the same number of objects. As an adult, this likely seems obvious – an implicit truth. But conservation is actually quite complicated and surprising. Take, for example, this week’s image:

No matter how you count these duckies – left to right, right to left, top to bottom, bottom to top, spiraling from the center, randomly hoping around – the last duckie as number 16 and there are a total of 16 duckies. Look at the purple duckie. Like most of the duckies, it gets a different number name in each of these ways of counting: 11 when counting left to right, 10 when counting top to bottom, and 3 when counting in a spiral. Each duckie’s number changes but the total number of duckies does not. How is that? Understanding conservation is serious cognitive work, which explains why children often need to count sets multiple times to be sure they understand how many there are and develop conservation as a stable attribute of counting.
Invite your students to count – and recount – and notice aloud that not matter where they start and end, there are always 16 duckies in this image.
And we invite you to follow us on Twitter! Tweet us the fascinating ideas you students have about our activities or how you’re trying these activities in your space. We can’t wait to hear from you!
To multiplicity, cheers!
Jen Munson and the multiplicity lab group